Sale Discount Equations Formulas Calculator

Sale Discount Coupons Shopping Saving Cost Percentage

Problem:

Solve for percent off.

Enter Calculator Inputs:

Can you share this page? Because, it could help others.

Solution:

Enter input values and press Calculate.

Change Equation or Formulas:

Tap or click to solve for a different unknown or equation

Percent Error Equation

	Solve for percent off or percentage.
	Solve for sale or discounted price.
	Solve for original or retail price
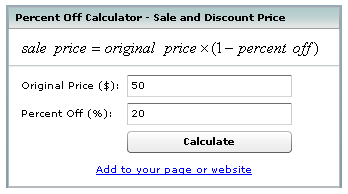	Rich Internet Application (RIA) Widget Percent off Calculator. Solve for sale or discount price.

Background

Calculating the percent off an item's sale price and original price is a fundamental math skill that is useful in various real-world situations—from understanding discounts during shopping to analyzing financial reports. It involves determining the percentage difference between an item's original price and the sale price. This skill is essential not only for consumers but also for professionals in several fields.

Equation

The equation to calculate the percent off is as follows:

Percent Off = (Original Price - Sale Price) / Original Price x 100 %

How to Solve

To solve for the percent off:

Deduct the sale price from the original price to find the discount amount.
Divide the discount amount by the original price. This gives you the discount fraction.
Multiply the discount fraction by 100 to convert it to a percentage. This is the percent off.

Example

Let's say a coat's original price is $80, but it's on sale for $56.

Deduct the sale price from the original price: $80 - $56 = $24.

Divide the discount amount by the original price: $24 / $80 = 0.3.

Multiply by 100 to find the percent off: 0.3 × 100 = 30%.

So, the coat is 30% off its original price.

Fields/Degrees It Is Used In

Marketing: Professionals calculate discounts and create pricing strategies.
Economics: Understanding market trends and the effect of pricing on demand.
Finance: Analysts use it to assess investment discounts or valuations.
Retail Management: To manage inventory and sales strategies.
Accounting: Helpful in preparing financial reports and budgeting.

Real-Life Applications

Shopping Discounts: Calculating how much you save on discounted items.
Couponing: Determining the value of coupons and promotional codes.
Budgeting: Estimating expenses by calculating expected discounts on goods.
Investing: Analyzing stock market discounts and premiums on securities.
Pricing Analysis: Businesses setting sale prices to attract customers.

Common Mistakes

Forgetting to Multiply by 100: Skipping this step results in a decimal instead of a percentage.
Mixing up the Original and Sale Price: Placing them incorrectly in the equation.
Rounding Errors: Early rounding can lead to inaccurate percent-off calculations.
Not Subtracting the Sale Price from the Original Price: This error will give you an incorrect discount amount.
Ignoring Context: Failing to consider buy-one-get-one deals or additional store discounts.

Frequently Asked Questions

What if the percent off is more than 100%?
This situation is theoretically impossible in retail, as it would mean the store would pay you to take the item. A percentage higher than 100% indicates a calculation mistake.
Can the sale price be higher than the original price?
Yes, in cases of pricing errors or if the item becomes more valuable over time. However, the "percent off" calculation is typically used to quantify discounts, not markups.
What do I do if I only know the percent off and the original price?
You can calculate the sale price by rearranging the percent-off formula: Sale Price = Original Price—(Original Price × Percent Off / 100).
How do I calculate the original price if I only know the sale price and percent off?
Rearrange the formula to solve for the original price:
Original Price = Sale Price / (1 - Percent Off / 100).
Is there a difference between 'percent off' and 'discount percent'?
No, they are often used interchangeably to describe how much the price of an item has been reduced in terms of percentage.

Percent Off Equations Formulas Calculator